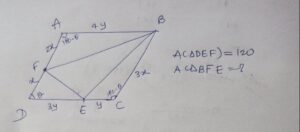
Q. In a parallelogram ABCD, points F and E are on AD and DC respectively. Point F divides AD in the ratio 2 : 1 and point E divides CD in the ratio 1 : 3. If the area of triangle DFE is 120 sq. units, then find the area (in sq units) of triangle BFE.

A. 480

B. 450

C. 400

D. 500

C. 400

Area of triangle DEF = 120

\(\frac{1}{2} \times x \times 3y \times \sin\theta = 120\)

⇒ xy sinθ = 80

Now, area of parallelogram ABCD = 3x × 4y × sinθ = 960

We know, sin(180 – θ) = sinθ

Area of triangle AFB = \(\frac{1}{2} \times 2x \times 4y \times \sin\left(180 – \theta \right) = 320\)

Area of triangle BCE = \(\frac{1}{2} \times 3x \times y \times \sin\left(180 – \theta \right) = 120\)

So, area of traingle BFE = 960 – [120 + 320 + 120] = 400